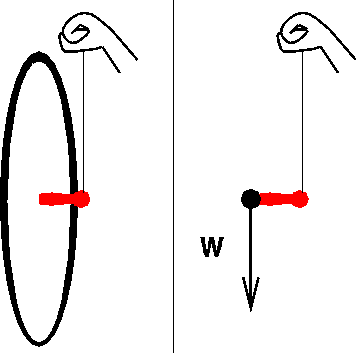
A gyroscope is a a fascinating mechanical device with a lot of technological applications. You can easily make a gyroscope from a wheel with a handle put through the middle.
If you dangle the end of the handle from a string,
the wheel doesn't flop over put stays up straight!
This might seem a bit odd because the weight 
is pointing straight
down. Instead it slowly moves around
in a circle, that is it precesses. Here's a picture of what's going on
when you look down on this from above. The wheel is shown at two different times.
I kid you not! This is quite counter-intuitive but can easily be understood by using the concepts of angular momentum and torque.
The angular momentum of the wheel points along its axis of rotation.
Here's the same picture but with the angular momentum vectors 
displayed.
Now let's think about what the angular momentum vectors do as a function of time.
Here they are displayed for two times t and t+dt. The difference between them
is the vector 
. Does this make sense yet? Well what direction is the
torque? As shown in the figure, it points in the horizontal plane. We
know that
So 
points in the same direction as . That's what we see in the
figure. He're we're assuming that the rate of rotation of the wheel 
is much greater than the rate of precession 
, so that we can
properly use 
. So let's compute the rate of precession.
If the length from the string to the center of mass of the wheel is called L, then the torque has magnitude
What is dL? It's 
. So
But by definition
So we have
The bigger , the slower the wheel precesses. The larger L, the distance between
the center of mass and the axis of rotation, the faster the object precesses.
Let's test how well we understand this
