Anomalous one dimensional dynamics

Also in collaboration with Onuttom Narayan, they have looked at thermal conductivity in one dimensional systems where various momentum and energy conserving models show that heat is transported much faster than one would naively expect. The thermal conductivity coefficient scales with a power of the system length L instead of being independent of system size, as happens in three dimensions. The value of the thermal conductivity predicted from Galilean invariance and the renormalization group is proportional to L^1/3. However this is difficult to verify numerically because finite size effects are unusually strong in such systems. 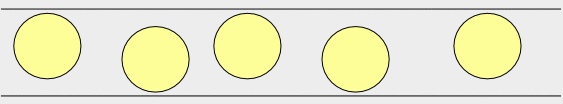Deutsch and Narayan employed two models, the Sinai Chernov "Pencase" model, pictured on the right. And a model they devised called the "Random Collision" model, which is very efficient numerically and allows one to get much further in to the asymptotic large L regime. Their worked verified the theoretical L^1/3 prediction.

The spatio-temporal behavior of correlation functions was also investigated and it was found 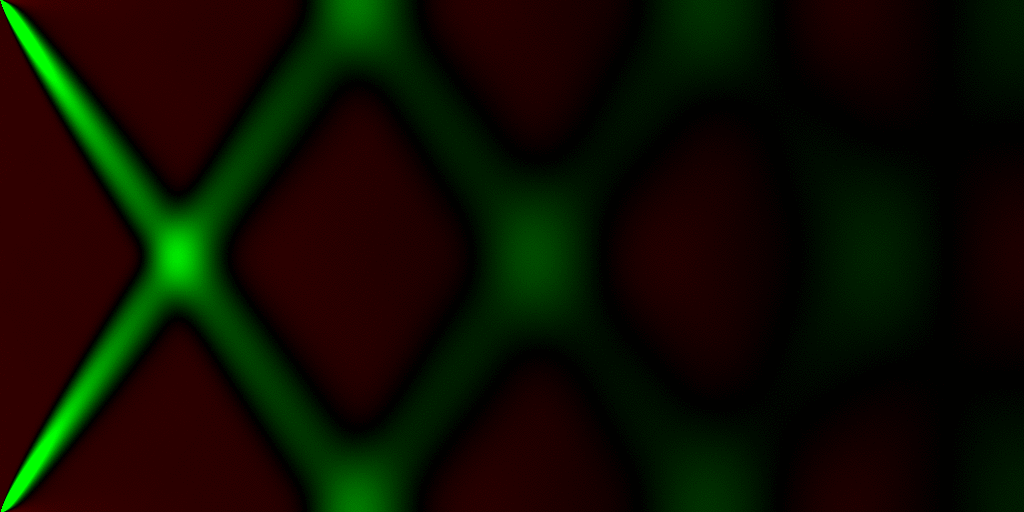that boundary conditions play a crucial role in such systems. To the right is an illustration of the velocity-velocity autocorrelation function, with time on the horizontal and position on the vertical axis. Periodic boundary conditions were used here. The green lines show a sound wave moving through the system. Note that it continues to be quite strong even after it has hit the boundary. The width of the line broadens is related to the relaxation of momentum and energy.