Professor Flipper, is explaining to the class the physics of friction. Despite the fact that he is a penguin, the audience is captivated by his eloquent oratory style.
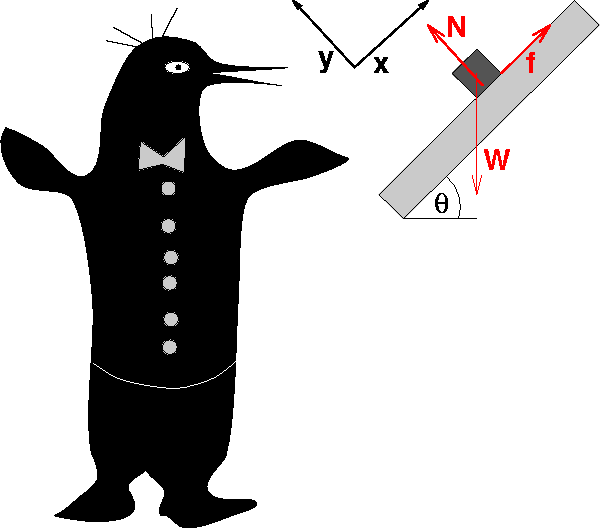
To demonstrate how it works, consider an inclined plane made out of
steel. Lets take this metal block and place it on the plane. The
plane makes an angle 
with the horizontal. When the plane
is flat, that is 
, the block sits on the plane, motionless.
Now lets increase a little. Interesting, the block stays there.
This is the force of friction at work. Without this force, any block
would accelerate down the inclined plane, but the force of friction,
as pictured above, applies just the right force to keep this block
motionless. Along the direction of the inclined plane, the force of
gravity has a component 
. If the acceleration in the x direction
is zero, then the net force must be zero by Newton's second law.
Therefore the force of friction must be exerting a force 
in
the x direction. Now what happens if we increase the angle some more?
will increase, but look!
The block continues to stay still. Therefore the frictional seems to
be equal to . Let's draw a graph of this:
Here we have the frictional force f as a function
of the applied force.
Along the red line the block is completely motionless
or static. Because the block isn't moving, this
is called static friction, and we'll denote the
force by the symbol 
. It's direction is along the
plane opposite to that of the applied force.
Now let's increase the angle just a smidgen more. Look,
the block is sliding down the inclined plane!
Notice it is not sliding freely, clearly the force of
friction is having a considerable effect on its motion
still. So in this case we'll call this friction
kinetic friction, 
, because it involves
an object in motion.
Let's draw in this part of the friction curve:
Here I've drawn the kinetic part in blue. It's been found experimentally to be pretty flat at low speeds and have close to what is shown.
Clearly we have a transition between two different kinds of behavior: sticking, and slipping. Let's draw the transition region in green:
What is the threshold for unsticking? That is, what is the maximum static friction force that can be sustained without the block giving way? Well countless brilliant humans and penguins have pondered this problem and have come up the formula relating it to the normal force N:
That is, the maximum static frictional force that
can be sustained is proportional to the strength
of the normal force. Now the coefficient of proportionality
is this symbol 
, which is often called the
coefficient of static friction. Normally it is a number
of around one or less, and depends on the nature of the two
surfaces. A shoe on a banana peel will be expected to
have a small coefficient of static friction, where as
Good running shoes are designed to have good traction
and hence a large coefficient of static friction.
How about the value of the kinetic friction, ?
Again this has been the subject of much cogitation
and the answer is
Again it's proportional to the normal force and the coefficient
of proportionality is called 
, the coefficient
of kinetic friction. Experimentally it appears that
.
Another miraculous thing about this is that these formulas only depend on the kind of material, not the surface area in contact. It kind of makes sense. If the surface area was small, like if you were wearing high heeled shoes instead of loafers, the pressure would be greater, so it would dig in to the ground more, thus compensating for less contact area.
So now I have elucidated the complete curve which is presented below:
The professor squawks, and the class sighs in awe at the miracle of what they've witnessed. Surely he must be the best penguin teacher on campus. But quite unexpectedly, a strange person walks in, wearing what appears to be an English policeman uniform, an ``English bobby''. He sits quietly down in the front row, and this seems to perturb the penguin, his voice becomes more bird-like but he continues with his lecture anyway. He squawks now more frequently.
Now let's figure out how to measure the coefficient of static We have the free body of this situation as shown:
The setup is identical to a previous example
see 1.5.1. There instead of a frictional
force, we had a string bearing a tension T, but
the effect is the same, to keep the block in place.
Eq. 1.9 says 
and
eq. 1.11 becomes 
.
At the angle where the block is about to slide, the frictional
force is given by eq. 1.16 and we therefore have
which says that 
.
Now let's demonstrate this. Ah, the block starts slipping right here at 31 degrees, if I'm not mistaken.
The penguin tries it again but this time it slips down at a different angle, 39 degrees
The curve the penguin drew figure 1.6 doesn't really exist. The green region in particular is bogus. It is not at all reproducible. It depends on whether you start with a static object and increase the applied force, or you start with a moving object and decreases the applied force. The physics of friction is a very interesting but complex subject and is currently under investigation by a lot of researchers, that are hopefully a good deal more honest than Professor Flipper.
