Microscopic origin of thermodynamic entropy

In quantum mechanics, it is useful to consider mix states which are statistical ensembles of pure states. These are most commonly described by the density matrix and Von Neumann has defined an entropy for these states. In the particular case of a pure state, where the wave function is known, the Von Neumann entropy is identically zero. The Von Neumann entropy is often referred to simply as the entropy, and this has led to the notion that the entropy of a pure state is zero.

In classical mechanics if the initial conditions of a system are completely known, is the entropy zero? In one sense it is, because the precise knowledge of particle positions and momenta allow for the extraction of work, through for example, the judicious placement of Maxwellian demons. However even if this is the case, the thermodynamic entropy still is measurable. The pressure exerted by a gas, and response to energy flowing into it, are not dependent on the experimenter's exact knowledge of the system and are derivable from knowing the thermodynamic entropy. Boltzmann's postulate for the entropy, being the logarithm of the volume of phase space that is eventually occupied by the system, is still well defined even for deterministic systems, and is central to understanding the a system's thermodynamics.

Is there an analog to this Boltzmann entropy in the quantum world? For example, will a system in an energy eigenstate possess the entropy normally expressed in terms of an ensemble description? Many papers have argued that it does not and the entropy is zero. However this comes from the same misconception that exists in the classical description for the system. Using the Eigenstate Thermalization Hypothesis Eigenstate Thermalization Hypothesis(ETH), this question can be analyzed theoretically and numerically. 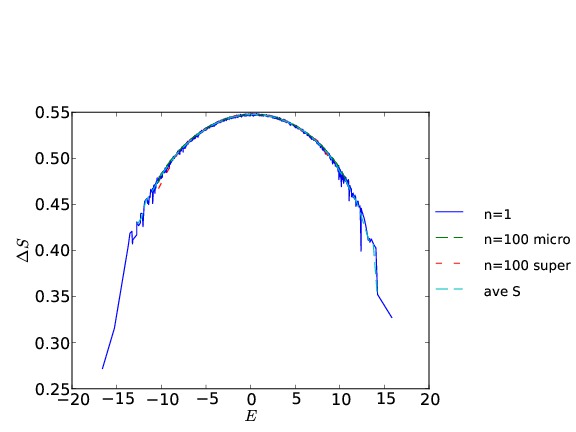Altogether, this provides strong evidence that the entanglement entropy of a generic quantum system in an energy eigenstate determines the thermodynamic entropy for the system. The figure shown here shows the entanglement entropy per site of a 6 hard core bosons on a 5 by 5 2d square lattice as a function of the energy. The answer obtained for a single energy eigenstate, n=1, is very close to what is found by doing a microcanonial ensemble average, over n=100 states. That ensemble average should be equivalent, in the thermodynamic limit, to the thermodynamic entropy by site. This equivalence breaks down if the system is integrable.

In many ways it is quite surprising that a state that is stationary in time, could give the same entropy as a typical state, where the time depedence will appear extremely chaotic. However an energy eigenstate at finite temperature is highly entangled with itself and this leads to thermodynamic behavior identical to what is seen in a mixed state.