Overdamping: $b^2 ~>~ 4mk$

In this case both roots of lambda are real.

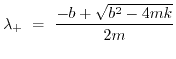$$\lambda_+ ~=~ {-b + \sqrt{b^2 -4mk}\over 2m}$$ (1.75)

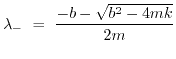$$\lambda_- ~=~ {-b - \sqrt{b^2 -4mk}\over 2m}$$ (1.76)

This means that both solutions decay exponentially

$$x(t) ~=~ \{e^{\lambda_+ t},e^{\lambda_- t}\}$$ (1.77)

Here is an example of such a decay, $x(t) = {1 \over 2}(\exp(-2t)+\exp(-0.5t))$.

Now as the damping $b$ increases, the two solutions $\lambda_+$ and $\lambda_-$ become very different. $\lambda_+ \rightarrow ~0$ while $\lambda_- \rightarrow \infty$ . When $\lambda_+$ is very small, that means the decay is very slow. So as you increase the friction $b$, the decay is slowed down. This is the opposite that happened in the above case of underdamping. We call this case overdamping because there are no oscillations, but the decay can be quite slow because the friction is so high that it's hard for the mass to move.

So let's ask the following question. What is the best value of the friction $b$ to choose so that the mass comes back to equilibrium most quickly. This is important if you were trying to design shock absorbers for a car. If $b$ is too small it just oscillates back and forth for a long time without decaying in amplitude much. If $b$ is too large, like in molasses, or tar, then it takes along time just to move the mass at all.

It turns out, that the best choice of $b$, is the critically damped case where $b^2 ~=~ 4mk$. It is at the point straddling the over and underdamped regimes. We won't solve this case but here is a plot of the way it looks

This plots the function $x(t) ~=~ \exp(-t)(1-t)$. The green line is a plot of $\exp(-t)$.